How to Understand Economic and Financial Crises Using the Tools from Far-From-Equilibrium Physics

Summary: I believe the key to managing financial crises is to understand the how systems behave when they are far from equilibrium. You can read more about the theoretical foundations of Far-From-Equilibriuim economics and finance by clicking here, which will take you to a downloadable PDF file of a recent article that I wrote for the Journal of Financial Economics. I hope that you enjoy the article.
Financial Crisis as Cascading Network Failure
In my last article I posted a set of graphs that illustrate a framework for understanding the sudden collapse of a robust economy into a financial crisis and the nature of its subsequent recovery. This piece is to provide a foundation for understanding the theory of financial crises embodied in the graphs. I believe it is a good basis for understanding the economic and financial impacts of the coronavirus crisis that is taking place today.
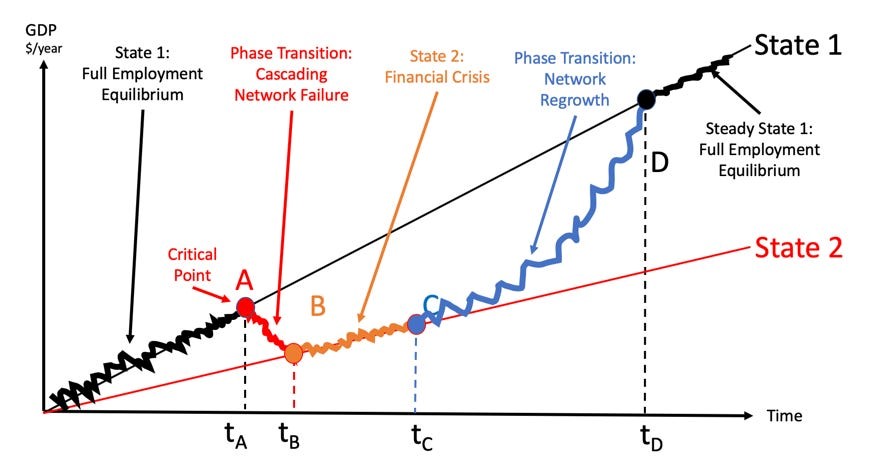
This framework views an economy at full employment as a smoothly functioning information network of the sort proposed almost 100 years ago by Friedrich von Hayek. It views the sudden onset of a financial crisis as a cascading network failure, or phase transition, to a less efficient state, something like what happens when an electricity grid fails. And it treats the gradual return to full employment as a second phase transition as the information network reconnects people over time. Unfortunately, in economics as well as nature, these phase transitions are unavoidable but to some degree they can be mitigated and managed.
This approach, known as Complex Adaptive Systems (CAS), is the result of thousands of scientific papers over the past thirty years in physics, chemistry, biology, and mathematics. In the document above, I describe the framework and summarize a number of the results. My hope is that it will help people understand and manage their way through the situations like we find ourselves in today.
I have been fascinated by the area since my daughter Jessica–also burdened by the math geek gene–introduced me to CAS and network theory 30 years ago. Since then, I have studied CAS, written about it, lectured on it at the Chinese Academy of Sciences, and taught a course in it every year to the PhD students at Claremont Graduate University. I believe it is the key to understanding one of the black holes in standard economic–why market economies can be both highly efficient most of the time and also subject to periodic, sudden financial crises. I will attach the syllabus for that course to my next post.
As you will see, this is a relatively nontechnical discussion of the ideas behind financial crises. The topic itself, however, is not nontechnical (pardon the double negative). It is based on a relatively new area in physics known as far-from-equilibrium thermodynamics, pioneered by Belgian chemist Ilya Prigogine (Nobel Prize in Chemistry, 1977) and others.
I won’t try to explain the subject matter here. You can get a good start on the ideas, as well as some of the most important references, in the article. Suffice it to say that the difference between near- and far-from-equilibrium change is like the difference between a gentle breeze and a hurricane. Or, as the late Per Bak wrote, “Almost all changes are small. Almost all changed is big.” I will write more on this subject this week.
Dr. John
The views and opinions expressed in this article are those of Dr. John Rutledge. Assumptions made in the analysis are not reflective of the position of any entity other than Dr. Rutledge’s. The information contained in this document does not constitute a solicitation, offer or recommendation to purchase or sell any particular security or investment product, or to engage in any particular strategy or in any transaction. You should not rely on any information contained herein in making a decision with respect to an investment. You should not construe the contents of this document as legal, business or tax advice and should consult with your own attorney, business advisor and tax advisor as to the legal, business, tax and related matters related hereto.
